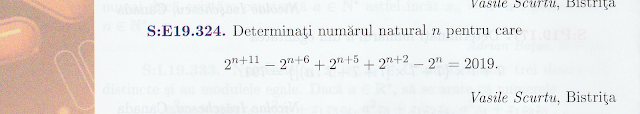The problem is proposed for the 5th grade, at page 6.
" Determine the natural number $n$ for which:
$$2^{n+11}-2^{n+6}+2^{n+5}+2^{n+2}-2^n=2019."$$
ANSWER CiP
$$n=0;$$
Indeed: $2^{11}-2^6+2^5+2^2-2^0= 2048-64+32+4-1=2019.$
Solution CiP
If the number $n>0$, then all the powers of $2$ on the left side of the equation are even numbers, so the result of their sum is an even number, so it cannot be equal to $2019.$
All that remains is to check the number $n=0$, and it is found that he verifies the equation.
$\blacksquare$
REMARKS CiP
$1^R$. We will use the following formula
$$2^k\;+\;2^k\;=\;2^{k+1},\;\;\;2^{k+1}-2^k=2^k,\;\;k=0,1,2,...$$
$2^R$. The number in base ten $2019$ is written in base $2$ as follows:
$2019_{(10)}=111\;1110\;0011_{(2)}$
$\Leftrightarrow\;\;2019=2^{10}+2^9+2^8+2^7+2^6+2^5+2^1+2^0.$
As it is known, this writing is unique, there is no one like it. Replacing the terms $2^k$ with the second formula in Remark $1^R$, with the exception of $2^5$, we have:
$2019=(2^{11}-2^{10})+(2^{10}-2^9)+(2^9-2^8)+(2^8-2^7)+(2^7-2^6)\;\underline{+2^5}\;+(2^2-2^1)+(2^1-2^0).$
If we open all the parentheses and keep in mind that $x-y+y=x,\;\;x,y,z \in \mathbb{N}$, we get:
$2019=2^{11}-2^6+2^5+2^2-2^0.$
$3^R$. The given equation can still be written, also with the formulas from the mentioned remark:
$2^{n+10}+2^{n+10}-2^{n+6}+2^{n+5}+2^{n+1}+2^{n+1}-2^n=2019$
$\Leftrightarrow\;2^{n+10}+(2^{n+10}-2^{n+9})+(2^{n+9}-2^{n+8})+(2^{n+8}-2^{n+7})+(2^{n+7}-2^{n+6})+2^{n+5}+(2^{n+2}-2^{n+1})+(2^{n+1}-2^n)=2019$
$\Leftrightarrow\;2^{n+10}+2^{n+9}+2^{n+8}+2^{n+7}+2^{n+6}+2^{n+5}+2^{n+1}+2^n=2019,$
and considering the unique writing in base $2$, we obtain the only possibility $n = 0.$
$\square\;\square\;\square$